مواد ڏانھن هلو

رياضي

ڳنڍڻن کي سنواريو
کليل ڄاڻ چيڪلي، وڪيپيڊيا مان

رياضي (mathematics) جو لفظ رياضت مان بڻيو آهي جنهنجو مطلب، سکڻ يا مشق ڪرڻ يا پڙهڻ ٿيندو آهي. رياضي جو علم اصل ۾، انگن جي استعمال جي ذريعي مقدارن جي خاصيتن ۽ ان جي وچ ۾ تعلقن جي تحقيق ۽ مطالعي کي چيو وڃي ٿو، ان کان علاوه ان ۾ ڍانچن، شڪلن ۽ تبدلات سان لاڳاپيل بحث بہ ڪيو وڃي ٿو. ھن علم جي باري ۾ گمان غالب آھي تہ ھن جي ابتدا يا ارتقاء دراصل ڳڻڻ (شمار ڪرڻ)، پئمائش ڪرڻ ۽ شين جي شڪلن ۽ حرڪتن جو مطالعو ڪرڻ جھڙن بنيادي عملن جي تجريد (abstraction) ۽ منطقي استدلال (logical reasoning) جي ذريعي ٿي.

رياضي دان، انھن تصورن ۽ فڪرن جي جيڪي مٿي ڏنل آھن، ڇنڊ ڇاڻ ڪندا آھن ۽ انھن سان لاڳاپيل بحث ڪندا آھن. ان جو مقصد نئين گمان ڪيل خيالن (conjecture) جي لاءِ صيغا (formula) اخذ ڪرڻ ھوندو آھي ۽ پوءِ احتياط سان چونڊيل مسلمات (axioms)، تعريفن ۽ قاعدن جي مدد سان رياضي جي اخذ ڪيل صيغن کي درست ثابت ڪرڻ آھي.

بنيادي قسم جي رياضي جي معلومات جو استعمال قديم زماني کان ئي مشھور آھي ۽ قديم مصر، بين النهرين ۽ قديم هندستان جي تھذيبن ۾ ان جا آثار ملن ٿا (ڏسو: رياضي جي تاريخ). اڄ دنيا ڀر ۾ علم رياضي سائنس، انجنيئرنگ، طب ۽ معاشيات سميت تمام علمي شعبن ۾ استعمال ڪيو پيو وڃي ۽ انھن اھم شعبن ۾ استعمال ٿيڻ واري رياضي کي عموماََ اطلاقي رياضي چيو وڃي ٿو تہ انھن شعبن تي رياضي جو نفاذ ڪري ۽ رياضي جي مدد وٺي ڪري نہ صرف نئين رياضياتي پھلوئن جي دريافت جا رستا کلجي وجهڻ ٿا، بلڪه، ڪڏهن ڪڏهن، رياضي ۽ ٻين شعبن جي انضمام يا ميلاپ سان، علم جا مڪمل طور تي نئين شعبي جي وجود ۾ اچڻ جا مثال موجود آهن.

تاريخي طور تي، ثبوت جو تصور ۽ ان سان لاڳاپيل رياضياتي سختي پهريون ڀيرو يوناني رياضي ۾ ظاهر ٿيو، خاص طور تي اقليدس (Euclide) جي عنصرن ۾. ان جي شروعات کان وٺي، رياضي کي بنيادي طور تي جاميٽري ۽ رياضي ۾ ورهايو ويو (قدرتي انگن ۽ جزن جي ڦيرڦار)، 16هين ۽ 17هين صدي تائين، جڏهن الجبرا ۽ ڪيلکولس کي نئين شعبن طور متعارف ڪرايو ويو. ان وقت کان وٺي، رياضياتي جدت ۽ سائنسي دريافتن جي وچ ۾ رابطي ٻنهي جي ترقي ۾ لاڳاپا اضافو ٿي چڪو آهي. 19هين صدي جي آخر ۾، رياضي جو بنيادي بحران محوري طريقي جي سسٽمائيزيشن جو سبب بڻيو، جنهن ۾ رياضياتي علائقن جي تعداد ۽ انهن جي استعمال جي شعبن ۾ هڪ ڊرامائي واڌ جو اعلان ڪيو. همعصر رياضيات جي مضمونن جي درجه بندي رياضي جي سٺ کان وڌيڪ پهرين-سطح جي علائقن کي لسٽ ڪري ٿي.

نالو

[سنواريو]

انگريزي ٻولي ۾ به ميٿميٽڪس (mathematics) جو لفظ يوناني ٻولي جي لفظ، ميٿيما (mathema) مان ماخوذ ڪيل آهي جنهن جو مطلب سکڻ يا پڙهڻ آهي.

رياضي جا ميدان

[سنواريو]

رينائسنس کان اڳ، رياضي ٻن مکيه علائقن ۾ ورهايل هئي: رياضي، انگن جي ڦيرڦار جي حوالي سان ۽ جاميٽري، شڪلن جي مطالعي جي حوالي سان.[1] ڪوڙي سائنس جا ڪي قسم، جهڙوڪ علم اعداد (Numerology) ۽ علم نجوم، ان وقت رياضي کان واضح طور تي فرق نه ڪيو ويو.[2]

ريناسنس دوران، ٻه وڌيڪ علائقا ظاهر ٿيا. رياضياتي اشارا الجبرا جو سبب بڻيو، جيڪو تقريبن فارمولن جي مطالعي ۽ ڦيرڦار تي مشتمل آهي. ڳڻپيوڪر (Caculus)، ٻن ذيلي شعبن تي مشتمل آهي فرق واري (Differential) ۽ انٽيگرل ڳڻپيوڪر (Integral Calculus) مسلسل ڪمن جو مطالعو آهي، جيڪي مختلف مقدارن جي وچ ۾ عام طور تي غير لڪيري لاڳاپن کي ماڊل ڪن ٿا، جيئن متغيرن مقدارن جي نمائندگي، متغير طور ڪن ٿا. هيءَ تقسيم چئن مکيه علائقن؛ رياضي، جاميٽري، الجبرا ۽ ڳڻپيوڪر، 19هين صدي جي آخر تائين برقرار رهي.[3] فلڪياتي ميڪانڪس ۽ سولڊ ميڪنڪس جهڙا علائقا ان وقت رياضي دان پڙهندا هئا، پر هاڻي انهن کي فزڪس سان لاڳاپيل سمجهيو وڃي ٿو.[4]ڪمبينيٽرونڪس (combinatorics) جو موضوع گهڻو ڪري رڪارڊ ٿيل تاريخن لاءِ مطالعو ڪيو ويو آهي، پر سترهين صديءَ تائين رياضي جي الڳ شاخ نه بڻجي سگهيو.[5]

19هين صدي جي آخر ۾، رياضي ۾ بنيادي بحران ۽ نتيجي ۾ محوري طريقي جي سسٽمائيزيشن رياضي جي نئين علائقن جي ڌماڪي جو سبب بڻيو.[6] سال 2020ع جي رياضي جي مضمون جي درجه بندي ۾ 63 کان گهٽ نه پهرين سطح وارا علائقا شامل آهن.[7] انهن علائقن مان ڪجهه پراڻن ڀاڱن سان ملندڙ جلندڙ آهن، جيئن انگن جو نظريو (اعليٰ رياضي جو جديد نالو) ۽ جاميٽري. پهرين سطح جي ٻين ڪيترن ئي علائقن جي نالن ۾ لفظ، ”جاميٽري“ شامل آهي يا ٻي صورت ۾ عام طور تي جاميٽري جو حصو سمجهيا وڃن ٿا. الجبرا ۽ ڳڻپيوڪر پهرين سطح جي علائقن جي طور تي ظاهر نه ٿيندا آهن پر ترتيب سان ڪيترن ئي پهرين سطح جي علائقن ۾ ورهايل آهن. 20هين صدي عيسويءَ دوران ٻيا پھرين سطح جا علائقا، جھڙوڪ رياضياتي منطق ۽ بنياد، اُڀريا يا اڳي انھن کي رياضي طور نه سمجھيو ويو ھو.[8]

انگن جو نظريو

[سنواريو]
اصل مضمون جي لاءِ ڏسو انڱن جو نظريو
هي الم اسپائرل آهي، جيڪو بنيادي نمبرن جي تقسيم کي بيان ڪري ٿو. اسپائرل ۾ ڳاڙهي ڊاريون لائينون نظرياتي اندازي مطابق، پرائم هجڻ ۽ ڪوڊراٽڪ پولينوميل جي قدر هجڻ جي وچ ۾، آزاديءَ تي اشارو ڪن ٿيون، هڪ اندازو جيڪا هاڻي هارڊي ۽ لٽل ووڊ جو ڪانجيڪٽر F سان سڃاڻو ويندو آهي.

انگن جو نظريو انگن جي ڦيرڦار سان شروع ٿيو، يعني قدرتي انگ (N) ۽ بعد ۾ انٽيجرز (Z) ۽ منطقي انگ (Q) تائين وڌايو ويو. انگ جي نظريي کي ڪنهن زماني ۾ رياضي سڏيو ويندو هو، پر اڄڪلهه اهو اصطلاح اڪثر ڪري عددي حسابن لاءِ استعمال ٿيندو آهي.[9] انگن جو نظريو قديم بابل ۽ شايد چين ڏانهن واپس اچي ٿو. ٻه نمايان ابتدائي نمبر جا نظريا دان قديم يونان جو اقليدس (Euclid) ۽ اسڪندريا، مصر جو ڊيوفانتس هئا.[10] انڱن جي نظريي جو جديد مطالعو ان جي تجريدي شڪل ۾ گهڻو ڪري پيئري ڊي فرمٽ ۽ ليون هارڊ ايولر ڏانهن منسوب ڪيو ويو آهي. ائڊرين-ميري ليجنڊري ۽ ڪارل فريڊرخ گاس جي مدد سان فيلڊ مڪمل طور تي ڪامياب ٿيو.[11]

ڪيتريون ئي آساني سان بيان ڪيل انگن اکرن جا مسئلن جا حل آهن جنھن کي، اڪثر ڪري رياضي کان نفيس طريقن جي ضرورت هونديون آهن. هڪ نمايان مثال فرمٽ جو آخري ٿيورم آهي. اهو اندازو 1637ع ۾ پيئر ڊي فرمٽ پاران بيان ڪيو ويو هو، پر اهو 1994ع ۾ اينڊريو وائلز طرفان ثابت ڪيو ويو، جنهن اسڪيم جي نظريي کان وٺي الجبرڪ جاميٽري، ڪيٽيگري ٿيوري ۽ هومولوجيڪل الجبرا جا اوزار استعمال ڪيا.[12] ٻيو مثال گولڊباخ جو اندازو آهي، جنهن ۾ اهو ثابت ڪري ٿو ته 2 کان وڏو هر انٽيجر ٻن بنيادي انگن جو مجموعو آهي. 1742ع ۾ ڪرسچن گولڊباخ پاران بيان ڪيو ويو، اهو اندازو ڪافي ڪوشش جي باوجود اڃا تائين ثابت نه ٿي سگهيو آهي.[13]

انگن جي نظريي ۾ ڪيترائي ذيلي علائقا، جن ۾ تجزياتي نمبر جو نظريو، الجبري نمبر جو نظريو، انگن جي جاميٽري (طريقه مبني)، ڊيوفانٽائن مساواتون ۽ عبوري نظريو (پرابلم اورينٽيڊ) شامل آھن.[14]

جاميٽري

[سنواريو]
اصل مضمون جي لاءِ ڏسو جاميٽري
هڪ گولي جي مٿاڇري تي، اقليدس جي جاميٽري صرف مقامي تقريبن جي طور تي لاڳو ٿئي ٿي. وڏي پيماني لاءِ ٽڪنڊي جي زاوين جو مجموعو °180 جي برابر نا هوندو هي.

جاميٽري رياضي جي قديم ترين شاخن مان هڪ آهي. ان جي شروعات تجرباتي شين سان ٿي جيڪا شڪلين جي حوالي سان ٿي، جيئن ته لائينون، زاويا ۽ دائرا، جيڪي خاص طور تي سروي ۽ فن تعمير جي ضرورتن لاءِ ٺاهيا ويا، پر ان کان پوءِ ڪيترن ئي ٻين ذيلي شعبن ۾ ترقي ڪري چڪو آهي. هڪ بنيادي جدت هئي قديم يونانين پاران ثبوتن جي تصور جو تعارف، جنهن جي ضرورت آهي ته هر دعوي کي ثابت ڪيو وڃي. مثال طور، ماپ جي ذريعي تصديق ڪرڻ ڪافي ناهي ته، چئو، ٻه ڊگھيون برابر آهن؛ انهن جي برابري کي ثابت ٿيڻ گهرجي استدلال جي ذريعي اڳوڻي قبول ٿيل نتيجن (نظريات) ۽ ڪجهه بنيادي بيانن مان. بنيادي بيان ثبوت جي تابع نه آهن ڇو ته اهي خود واضح آهن (موضوعات)، يا مطالعي جي موضوع جي تعريف جو حصو آهن (محور). هي اصول، سڀني رياضيات لاءِ بنيادي، پهريون ڀيرو جاميٽري لاءِ وضاحت ڪئي وئي هئي، ۽ 300 ق. نتيجي ۾ نڪرندڙ ايڪليڊين جاميٽري شڪلين جو مطالعو آهي ۽ انهن جي ترتيبن کي اڪيليڊن جهاز (جهاز جي جاميٽري) ۽ ٽي-dimensional Euclidean اسپيس ۾ لائينن، جهازن ۽ دائرن مان ٺهيل آهي. Euclidean جاميٽري 17 صدي عيسويء تائين طريقن يا دائري ۾ تبديلي جي بغير ترقي ڪئي وئي، جڏهن ريني ڊيڪارٽ متعارف ڪرايو جنهن کي هاڻي ڪارٽسين ڪوآرڊينيٽ سڏيو ويندو آهي. هن پيراڊم جي هڪ وڏي تبديلي کي قائم ڪيو: حقيقي انگن کي لڪير جي حصن جي ڊيگهه جي طور تي بيان ڪرڻ جي بدران، اهو انهن جي همراهن کي استعمال ڪندي پوائنٽن جي نمائندگي ڪرڻ جي اجازت ڏني، جيڪي انگ آهن. الجبرا (۽ بعد ۾، حساب ڪتاب) اهڙيء طرح جاميٽري مسئلن کي حل ڪرڻ لاء استعمال ڪري سگهجي ٿو.

is one of the oldest branches of mathematics. It started with empirical recipes concerning shapes, such as lines, angles and circles, which were developed mainly for the needs of surveying and architecture, but has since blossomed out into many other subfields.[15]

A fundamental innovation was the ancient Greeks' introduction of the concept of proofs, which require that every assertion must be proved. For example, it is not sufficient to verify by measurement that, say, two lengths are equal; their equality must be proven via reasoning from previously accepted results (theorems) and a few basic statements. The basic statements are not subject to proof because they are self-evident (postulates), or are part of the definition of the subject of study (axioms). This principle, foundational for all mathematics, was first elaborated for geometry, and was systematized by Euclid around 300 BC in his book Elements.[16][17]

The resulting Euclidean geometry is the study of shapes and their arrangements constructed from lines, planes and circles in the Euclidean plane (plane geometry) and the three-dimensional Euclidean space.[lower-alpha 1][15]

Euclidean geometry was developed without change of methods or scope until the 17th century, when René Descartes introduced what is now called Cartesian coordinates. This constituted a major change of paradigm: Instead of defining real numbers as lengths of line segments (see number line), it allowed the representation of points using their coordinates, which are numbers. Algebra (and later, calculus) can thus be used to solve geometrical problems. Geometry was split into two new subfields: synthetic geometry, which uses purely geometrical methods, and analytic geometry, which uses coordinates systemically.[18]

Analytic geometry allows the study of curves unrelated to circles and lines. Such curves can be defined as the graph of functions, the study of which led to differential geometry. They can also be defined as implicit equations, often polynomial equations (which spawned algebraic geometry). Analytic geometry also makes it possible to consider Euclidean spaces of higher than three dimensions.[15]

In the 19th century, mathematicians discovered non-Euclidean geometries, which do not follow the parallel postulate. By questioning that postulate's truth, this discovery has been viewed as joining Russell's paradox in revealing the foundational crisis of mathematics. This aspect of the crisis was solved by systematizing the axiomatic method, and adopting that the truth of the chosen axioms is not a mathematical problem.[19][20] In turn, the axiomatic method allows for the study of various geometries obtained either by changing the axioms or by considering properties that do not change under specific transformations of the space.[21]

Today's subareas of geometry include:[22]

Algebra

[سنواريو]
اصل مضمون جي لاءِ ڏسو Algebra
refer to caption
The quadratic formula, which concisely expresses the solutions of all quadratic equations
A shuffled 3x3 rubik's cube
The Rubik's Cube group is a concrete application of group theory.[23]

Algebra is the art of manipulating equations and formulas. Diophantus (3rd century) and al-Khwarizmi (9th century) were the two main precursors of algebra.[24][25] Diophantus solved some equations involving unknown natural numbers by deducing new relations until he obtained the solution.[26] Al-Khwarizmi introduced systematic methods for transforming equations, such as moving a term from one side of an equation into the other side.[27] The term algebra is derived from the Arabic word al-jabr meaning 'the reunion of broken parts' that he used for naming one of these methods in the title of his main treatise.[28][29]

Algebra became an area in its own right only with François Viète (1540–1603), who introduced the use of variables for representing unknown or unspecified numbers.[30] Variables allow mathematicians to describe the operations that have to be done on the numbers represented using mathematical formulas.[31]

Until the 19th century, algebra consisted mainly of the study of linear equations (presently linear algebra), and polynomial equations in a single unknown, which were called algebraic equations (a term still in use, although it may be ambiguous). During the 19th century, mathematicians began to use variables to represent things other than numbers (such as matrices, modular integers, and geometric transformations), on which generalizations of arithmetic operations are often valid.[32] The concept of algebraic structure addresses this, consisting of a set whose elements are unspecified, of operations acting on the elements of the set, and rules that these operations must follow. The scope of algebra thus grew to include the study of algebraic structures. This object of algebra was called modern algebra or abstract algebra, as established by the influence and works of Emmy Noether.[33]

Some types of algebraic structures have useful and often fundamental properties, in many areas of mathematics. Their study became autonomous parts of algebra, and include:[22]

The study of types of algebraic structures as mathematical objects is the purpose of universal algebra and category theory.[34] The latter applies to every mathematical structure (not only algebraic ones). At its origin, it was introduced, together with homological algebra for allowing the algebraic study of non-algebraic objects such as topological spaces; this particular area of application is called algebraic topology.[35]

Calculus and analysis

[سنواريو]
اصل مضمون/مضمونن جي لاءِ ڏسو Calculus ۽ Mathematical analysis
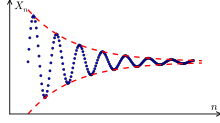
A Cauchy sequence consists of elements such that all subsequent terms of a term become arbitrarily close to each other as the sequence progresses (from left to right).

Calculus, formerly called infinitesimal calculus, was introduced independently and simultaneously by 17th-century mathematicians Newton and Leibniz.[36] It is fundamentally the study of the relationship of variables that depend on each other. Calculus was expanded in the 18th century by Euler with the introduction of the concept of a function and many other results.[37] Presently, "calculus" refers mainly to the elementary part of this theory, and "analysis" is commonly used for advanced parts.[38]

Analysis is further subdivided into real analysis, where variables represent real numbers, and complex analysis, where variables represent complex numbers. Analysis includes many subareas shared by other areas of mathematics which include:[22]

Discrete mathematics

[سنواريو]
اصل مضمون جي لاءِ ڏسو Discrete mathematics
A diagram representing a two-state Markov chain. The states are represented by 'A' and 'E'. The numbers are the probability of flipping the state.

Discrete mathematics, broadly speaking, is the study of individual, countable mathematical objects. An example is the set of all integers.[39] Because the objects of study here are discrete, the methods of calculus and mathematical analysis do not directly apply.[lower-alpha 2] Algorithmsسانچو:Emdashespecially their implementation and computational complexityسانچو:Emdashplay a major role in discrete mathematics.[40]

The four color theorem and optimal sphere packing were two major problems of discrete mathematics solved in the second half of the 20th century.[41] The P versus NP problem, which remains open to this day, is also important for discrete mathematics, since its solution would potentially impact a large number of computationally difficult problems.[42]

Discrete mathematics includes:[22]

Mathematical logic and set theory

[سنواريو]
اصل مضمون/مضمونن جي لاءِ ڏسو Mathematical logic ۽ Set theory
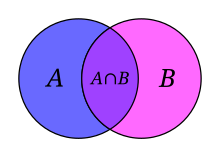
A blue and pink circle and their intersection labeled
The Venn diagram is a commonly used method to illustrate the relations between sets.

The two subjects of mathematical logic and set theory have belonged to mathematics since the end of the 19th century.[43][44] Before this period, sets were not considered to be mathematical objects, and logic, although used for mathematical proofs, belonged to philosophy and was not specifically studied by mathematicians.[45]

Before Cantor's study of infinite sets, mathematicians were reluctant to consider actually infinite collections, and considered infinity to be the result of endless enumeration. Cantor's work offended many mathematicians not only by considering actually infinite sets[46] but by showing that this implies different sizes of infinity, per Cantor's diagonal argument. This led to the controversy over Cantor's set theory.[47] In the same period, various areas of mathematics concluded the former intuitive definitions of the basic mathematical objects were insufficient for ensuring mathematical rigour.[48]

This became the foundational crisis of mathematics.[49] It was eventually solved in mainstream mathematics by systematizing the axiomatic method inside a formalized set theory. Roughly speaking, each mathematical object is defined by the set of all similar objects and the properties that these objects must have.[50] For example, in Peano arithmetic, the natural numbers are defined by "zero is a number", "each number has a unique successor", "each number but zero has a unique predecessor", and some rules of reasoning.[51] This mathematical abstraction from reality is embodied in the modern philosophy of formalism, as founded by David Hilbert around 1910.[52]

The "nature" of the objects defined this way is a philosophical problem that mathematicians leave to philosophers, even if many mathematicians have opinions on this nature, and use their opinionسانچو:Emdashsometimes called "intuition"سانچو:Emdashto guide their study and proofs. The approach allows considering "logics" (that is, sets of allowed deducing rules), theorems, proofs, etc. as mathematical objects, and to prove theorems about them. For example, Gödel's incompleteness theorems assert, roughly speaking that, in every consistent formal system that contains the natural numbers, there are theorems that are true (that is provable in a stronger system), but not provable inside the system.[53] This approach to the foundations of mathematics was challenged during the first half of the 20th century by mathematicians led by Brouwer, who promoted intuitionistic logic, which explicitly lacks the law of excluded middle.[54][55]

These problems and debates led to a wide expansion of mathematical logic, with subareas such as model theory (modeling some logical theories inside other theories), proof theory, type theory, computability theory and computational complexity theory.[22] Although these aspects of mathematical logic were introduced before the rise of computers, their use in compiler design, formal verification, program analysis, proof assistants and other aspects of computer science, contributed in turn to the expansion of these logical theories.[56]

شماريات ۽ ٻيا فيصلي جي سائنسون

[سنواريو]
اصل مضمون/مضمونن جي لاءِ ڏسو شماريات ۽ امڪان جو نظريو
بي ترتيب آباديءَ جي ورڇ (μ) جو جيڪو به روپ هجي، نمونن جو مطلب (x̄) گاس جي ورهائڻ (Gaussian distribution) ڏانهن هوندو آهي ۽ ان جو ويرينس (σ) امڪاني نظريي جي مرڪزي حد ٿيوريم طرفان ڏنو ويندو آهي.[57]

شماريات (انگن اکرن) جو ميدان هڪ رياضياتي ايپليڪيشن آهي جيڪو ڊيٽا جي نمونن جي گڏ ڪرڻ ۽ پروسيسنگ لاء، رياضياتي طريقن جي بنياد تي، خاص طور تي امڪاني نظريي جو طريقيڪار استعمال ڪندي استعمال ڪيو ويندو آهي. شماريات جا ماهر بي ترتيب نموني يا بي ترتيب ڪيل تجربن سان ڊيٽا ٺاهيندا آهن.[58]

شمارياتي نظريو مطالعي جي فيصلي جي مسئلن جهڙوڪ شمارياتي عمل جي خطري (متوقع نقصان) کي گھٽائڻ، جهڙوڪ طريقيڪار استعمال ڪندي، مثال طور، پيٽرولر تخميني، فرضي جاچ ۽ بهترين چونڊيو. رياضياتي انگن اکرن جي انهن روايتي علائقن ۾، هڪ شمارياتي-فيصلو وارو مسئلو هڪ مقصدي فنڪشن کي گھٽائڻ، جهڙوڪ متوقع نقصان يا قيمت، مخصوص رڪاوٽن جي تحت ٺاهيو ويندو آهي. مثال طور، هڪ سروي کي ڊزائين ڪرڻ ۾ اڪثر ڪري آبادي جو اندازو لڳائڻ جي قيمت کي گھٽائڻ شامل آهي مطلب هڪ ڏنل سطح جي اعتماد سان. ان جي اصلاح جي استعمال جي ڪري، انگن اکرن جو رياضياتي نظريو ٻين فيصلن جي سائنسن، جهڙوڪ آپريشن ريسرچ، ڪنٽرول ٿيوري، ۽ رياضياتي معاشيات سان اوورليپ ٿئي ٿو.

Statistical theory studies

decision problems such as minimizing the risk (expected loss) of a statistical action, such as using a procedure in, for example, parameter estimation, hypothesis testing, and selecting the best. In these traditional areas of mathematical statistics, a statistical-decision problem is formulated by minimizing an objective function, like expected loss or cost, under specific constraints. For example, designing a survey often involves minimizing the cost of estimating a population mean with a given level of confidence.[59] Because of its use of optimization, the mathematical theory of statistics overlaps with other decision sciences, such as operations research, control theory, and mathematical economics.[60]

حسابي رياضي

[سنواريو]
اصل مضمون جي لاءِ ڏسو حسابي رياضي

شمارياتي رياضيات رياضياتي مسئلن جو مطالعو آهي جيڪي عام طور تي انساني، عددي صلاحيت لاء تمام وڏا آهن.[61] [62] عددي تجزيي مطالعي جا طريقا تجزيي ۾ مسئلن لاء فنڪشنل تجزيو ۽ تقريبن نظريي کي استعمال ڪندي؛ انگن اکرن جي تجزيي ۾ وسيع طور تي ۽ خاص ڌيان سان گولن جي غلطين تي، تقريبات جو مطالعو شامل آهي.[63] عددي تجزيي ۽، وڌيڪ وسيع طور تي، سائنسي ڪمپيوٽنگ پڻ رياضياتي سائنس جي غير تجزياتي موضوعن جو مطالعو ڪري ٿو، خاص طور تي الگورٿمڪ-ميٽرڪس-۽-گراف ٿيوري. ڪمپيوٽري رياضي جي ٻين علائقن ۾ ڪمپيوٽر جي الجبرا ۽ علامتي حساب شامل آهن.

تاريخ

[سنواريو]

علامتي اشارا ۽ اصطلاحون

[سنواريو]

سائنس سان تعلق

[سنواريو]

علم نجوم ۽ باطنيات سان تعلق

[سنواريو]

فلسفو

[سنواريو]

تربيت ۽ مشق

[سنواريو]

ثقافتي اثر

[سنواريو]

ايوارڊ ۽ انعامي مسئلا

[سنواريو]
مرڪزي زمرو: رياضي جا انعام
فيلڊس ميڊل جي سامهون واري پاسي يوناني پولي ميٿ آرشيميدس جي تصوير سان

رياضي ۾ سڀ کان وڌيڪ معزز انعام فيلڊز ميڊل آهي، جيڪو سال 1936ع ۾ قائم ٿيو ۽ هر چئن سالن ۾ (سواءِ عالمي جنگ II جي چوڌاري) چئن ماڻهن تائين ڏنو ويو آهي. اهو نوبل انعام جي رياضياتي برابر سمجهيو ويندو آهي.

ٻين معزز رياضي انعامن ۾ شامل آهن:

* ايبل انعام، 2002ع ۾ قائم ڪيو ويو ۽ پهريون ڀيرو 2003ع ۾ نوازيو ويو.

* چرن ميڊل لائف ٽائيم اچيومينٽ سال 2009ع ۾ متعارف ڪرايو ۽ پهريون ڀيرو 2010ع ۾ انعام ڏنو ويو.

  • رياضي ۾ وولف انعام، تاحيات حاصلات لاء سال 1970ع ۾ قائم ڪيو ويو. سال 1978ع ليروئي پي. اسٽيلي کي انعام کان نوازيو ويو.

23 کليل مسئلن جي هڪ مشهور فهرست، جنهن کي "هلبرٽ جا مسئلا" سڏيو ويندو آهي، جرمن رياضي دان ڊيوڊ هيلبرٽ سال 1900ع ۾ مرتب ڪيو هو. ھن لسٽ رياضي دانن جي وچ ۾ وڏي شهرت حاصل ڪئي آھي ۽ گھٽ ۾ گھٽ تيرھن مسئلا (انحصار ڪن ٿا ته ڪھڙي ريت بيان ڪيا ويا آھن) حل ڪيا ويا آھن. ستن اهم مسئلن جي هڪ نئين فهرست، جنهن جو عنوان آهي "ملينيم پرائز پرابلمس"، سال 2000ع ۾ شايع ڪيو ويو. انهن مان صرف هڪ، ريمن جي نظريي، هيلبرٽ جي مسئلن مان هڪ کي نقل ڪري ٿو. انهن مان ڪنهن به مسئلي جو حل هڪ ملين ڊالر جو انعام آهي. اڄ تائين، انهن مسئلن مان صرف هڪ، "پوائنڪيري جو اندازو"، روسي رياضي دان گريگوري پيرلمين طرفان حل ڪيو ويو آهي.

most prestigious award in mathematics is the Fields Medal,[64][65] established in 1936 and awarded every four years (except around World War II) to up to four individuals.[66][67] It is considered the mathematical equivalent of the Nobel Prize.[67]

Other prestigious mathematics awards include:[68]

A famous list of 23 open problems, called "Hilbert's problems", was compiled in 1900 by German mathematician David Hilbert.[76] This list has achieved great celebrity among mathematicians,[77] and at least thirteen of the problems (depending how some are interpreted) have been solved.[76]

A new list of seven important problems, titled the "Millennium Prize Problems", was published in 2000. Only one of them, the Riemann hypothesis, duplicates one of Hilbert's problems. A solution to any of these problems carries a 1 million dollar reward.[78] To date, only one of these problems, the Poincaré conjecture, has been solved by the Russian mathematician Grigori Perelman.[79]

پڻ ڏسو

[سنواريو]
نشان بابباب رياضي
  • رياضي جي تاريخ
  • رياضياتي اصطلاحن جي فهرست
  • رياضي دانن جي فهرست
  • رياضي جي مضمونن جي فهرست
  • رياضياتي مستقل
  • رياضياتي سائنس
  • رياضي ۽ فن
  • رياضي جي تعليم
  • رياضي جو فلسفو
  • رياضي ۽ فزڪس جي وچ ۾ تعلق
  • سائنس، ٽيڪنالاجي، انجنيئرنگ ۽ رياضي
Search Wikimedia Commons وڪيميڊيا العام ۾ رياضي سان لاڳاپيل ابلاغي مواد ڏسو.

خارجي ڳنڍڻا

[سنواريو]
لائبريري وسيلا بابت
Mathematics

سانچو:Areas of mathematics

باب
لاڳاپيل موضوعن تائين رسائي
Mathematics باب
Arithmetic باب
History of science باب
Science باب
Find out more on Wikipedia's
ٻيا منصوبا
ميڊيا
from ڪامنز
خبرون
from وڪي خبرون
تعريفون
from وڪي لغت
نصابي ڪتاب
from وڪي ڪتاب
وڪي قول
from وڪي قول
وڪي وسيلا
from وڪي وسيلا
تعليمي مواد
from وڪيورسٽي

حوالا

[سنواريو]
  1. ↑ Bell, E. T. (1945). "General Prospectus". The Development of Mathematics (2nd ed.). Dover Publications. p. 3. ISBN 978-0-486-27239-9. OCLC 523284. "... mathematics has come down to the present by the two main streams of number and form. The first carried along arithmetic and algebra, the second, geometry." 
  2. ↑ Bell, E. T. (1945). "General Prospectus". The Development of Mathematics (2nd ed.). Dover Publications. p. 3. ISBN 978-0-486-27239-9. OCLC 523284. "... mathematics has come down to the present by the two main streams of number and form. The first carried along arithmetic and algebra, the second, geometry." 
  3. ↑ Restivo, Sal (1992). "Mathematics from the Ground Up". in Bunge, Mario. Mathematics in Society and History. Episteme. 20. Kluwer Academic Publishers. p. 14. ISBN 0-7923-1765-3. OCLC 92013695. 
  4. ↑ Musielak, Dora (2022). Leonhard Euler and the Foundations of Celestial Mechanics. History of Physics. Springer International Publishing. doi:10.1007/978-3-031-12322-1. ISBN 978-3-031-12321-4. OCLC 1332780664. 
  5. ↑ Biggs, N. L. (May 1979). "The roots of combinatorics". Historia Mathematica 6 (2): 109–136. doi:10.1016/0315-0860(79)90074-0. ISSN 0315-0860. OCLC 2240703. 
  6. ↑ Warner, Evan. "Splash Talk: The Foundational Crisis of Mathematics" (PDF). Columbia University. وقت March 22, 2023 تي اصل (PDF) کان آرڪائيو ٿيل. حاصل ڪيل February 3, 2024.  Unknown parameter |url-status= ignored (مدد)
  7. ↑ Dunne, Edward; Hulek, Klaus (March 2020). "Mathematics Subject Classification 2020". Notices of the American Mathematical Society 67 (3): 410–411. doi:10.1090/noti2052. ISSN 0002-9920. OCLC 1480366. https://www.ams.org/journals/notices/202003/rnoti-p410.pdf. Retrieved February 3, 2024. "The new MSC contains 63 two-digit classifications, 529 three-digit classifications, and 6,006 five-digit classifications.". 
  8. ↑ "MSC2020-Mathematics Subject Classification System" (PDF). zbMath. Associate Editors of Mathematical Reviews and zbMATH. وقت January 2, 2024 تي اصل (PDF) کان آرڪائيو ٿيل. حاصل ڪيل February 3, 2024.  Unknown parameter |url-status= ignored (مدد)
  9. ↑ LeVeque, William J. (1977). "Introduction". Fundamentals of Number Theory. Addison-Wesley Publishing Company. pp. 1–30. ISBN 0-201-04287-8. OCLC 3519779. 
  10. ↑ Goldman, Jay R. (1998). "The Founding Fathers". The Queen of Mathematics: A Historically Motivated Guide to Number Theory. Wellesley, MA: A K Peters. pp. 2–3. doi:10.1201/9781439864623. ISBN 1-56881-006-7. OCLC 30437959. 
  11. ↑ Weil, André (1983). Number Theory: An Approach Through History From Hammurapi to Legendre. Birkhäuser Boston. pp. 2–3. doi:10.1007/978-0-8176-4571-7. ISBN 0-8176-3141-0. OCLC 9576587. 
  12. ↑ Kleiner, Israel (March 2000). "From Fermat to Wiles: Fermat's Last Theorem Becomes a Theorem". Elemente der Mathematik 55 (1): 19–37. doi:10.1007/PL00000079. ISSN 0013-6018. OCLC 1567783. 
  13. ↑ Wang, Yuan (2002). The Goldbach Conjecture. Series in Pure Mathematics. 4 (2nd ed.). World Scientific. pp. 1–18. doi:10.1142/5096. ISBN 981-238-159-7. OCLC 51533750. 
  14. ↑ "MSC2020-Mathematics Subject Classification System" (PDF). zbMath. Associate Editors of Mathematical Reviews and zbMATH. وقت January 2, 2024 تي اصل (PDF) کان آرڪائيو ٿيل. حاصل ڪيل February 3, 2024.  Unknown parameter |url-status= ignored (مدد)
  15. ↑ 15.0 15.1 15.2 سانچو:Cite arXiv
  16. ↑ Hilbert, David (1902). [[[:سانچو:GBurl]] The Foundations of Geometry]. Open Court Publishing Company. p. 1. doi:10.1126/science.16.399.307. OCLC 996838. سانچو:GBurl. Retrieved February 6, 2024.  سانچو:Free access
  17. ↑ Hartshorne, Robin (2000). "[[[:سانچو:GBurl]] Euclid's Geometry]". Geometry: Euclid and Beyond. Springer New York. pp. 9–13. ISBN 0-387-98650-2. OCLC 42290188. سانچو:GBurl. Retrieved February 7, 2024. 
  18. ↑ Boyer, Carl B. (2004). "Fermat and Descartes". History of Analytic Geometry. Dover Publications. pp. 74–102. ISBN 0-486-43832-5. OCLC 56317813. 
  19. ↑ Stump, David J. (1997). "Reconstructing the Unity of Mathematics circa 1900". Perspectives on Science 5 (3): 383–417. doi:10.1162/posc_a_00532. ISSN 1063-6145. OCLC 26085129. https://philpapers.org/archive/STURTU.pdf. Retrieved February 8, 2024. 
  20. ↑ حوالي جي چڪ: Invalid <ref> tag; no text was provided for refs named Kleiner_1991
  21. ↑ O'Connor, J. J.; Robertson, E. F. "Non-Euclidean geometry". MacTuror. Scotland, UK: University of St. Andrews. وقت November 6, 2022 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل February 8, 2024.  Unknown parameter |url-status= ignored (مدد)
  22. ↑ 22.0 22.1 22.2 22.3 22.4 "MSC2020-Mathematics Subject Classification System" (PDF). zbMath. Associate Editors of Mathematical Reviews and zbMATH. وقت January 2, 2024 تي اصل (PDF) کان آرڪائيو ٿيل. حاصل ڪيل February 3, 2024.  Unknown parameter |url-status= ignored (مدد)
  23. ↑ Joyner, David (2008). "The (legal) Rubik's Cube group". Adventures in Group Theory: Rubik's Cube, Merlin's Machine, and Other Mathematical Toys (2nd ed.). Johns Hopkins University Press. pp. 219–232. ISBN 978-0-8018-9012-3. OCLC 213765703. 
  24. ↑ Christianidis, Jean; Oaks, Jeffrey (May 2013). "Practicing algebra in late antiquity: The problem-solving of Diophantus of Alexandria". Historia Mathematica 40 (2): 127–163. doi:10.1016/j.hm.2012.09.001. ISSN 0315-0860. OCLC 2240703. 
  25. ↑ Kleiner 2007, "History of Classical Algebra" pp. 3–5.
  26. ↑ Shane, David. "Figurate Numbers: A Historical Survey of an Ancient Mathematics" (PDF). Methodist University. صفحو. 20. حاصل ڪيل June 13, 2024. In his work, Diophantus focused on deducing the arithmetic properties of figurate numbers, such as deducing the number of sides, the different ways a number can be expressed as a figurate number, and the formulation of the arithmetic progressions. 
  27. ↑ Overbay, Shawn; Schorer, Jimmy; Conger, Heather. "Al-Khwarizmi". University of Kentucky. حاصل ڪيل June 13, 2024. 
  28. ↑ Lim, Lisa. "Where the x we use in algebra came from, and the X in Xmas". South China Morning Post. وقت December 22, 2018 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل February 8, 2024.  Unknown parameter |url-status= ignored (مدد); Unknown parameter |url-access= ignored (مدد)
  29. ↑ Berntjes, Sonja. "Algebra". Encyclopaedia of Islam Online. ISSN 1573-3912. LCCN 2007238847. OCLC 56713464. حاصل ڪيل June 13, 2024. 
  30. ↑ Oaks, Jeffery A. (2018). "François Viète's revolution in algebra". Archive for History of Exact Sciences 72 (3): 245–302. doi:10.1007/s00407-018-0208-0. ISSN 0003-9519. OCLC 1482042. https://researchoutreach.org/wp-content/uploads/2019/02/Jeffrey-Oaks.pdf. Retrieved February 8, 2024. 
  31. ↑ "Variable in Maths". GeeksforGeeks. حاصل ڪيل June 13, 2024. 
  32. ↑ Kleiner 2007, "History of Linear Algebra" pp. 79–101.
  33. ↑ Corry, Leo (2004). "[[[:سانچو:GBurl]] Emmy Noether: Ideals and Structures]". Modern Algebra and the Rise of Mathematical Structures (2nd revised ed.). Germany: Birkhäuser Basel. pp. 247–252. ISBN 3-7643-7002-5. OCLC 51234417. سانچو:GBurl. Retrieved February 8, 2024. 
  34. ↑ Riche, Jacques (2007). "[[[:سانچو:GBurl]] From Universal Algebra to Universal Logic]". in Beziau, J. Y.; Costa-Leite, Alexandre. Perspectives on Universal Logic. Milano, Italy: Polimetrica International Scientific Publisher. pp. 3–39. ISBN 978-88-7699-077-9. OCLC 647049731. سانچو:GBurl. Retrieved February 8, 2024. 
  35. ↑ Krömer, Ralph (2007). [[[:سانچو:GBurl]] Tool and Object: A History and Philosophy of Category Theory]. Science Networks – Historical Studies. 32. Germany: Springer Science & Business Media. pp. xxi–xxv, 1–91. ISBN 978-3-7643-7523-2. OCLC 85242858. سانچو:GBurl. Retrieved February 8, 2024. 
  36. ↑ Guicciardini, Niccolo (2017). "The Newton–Leibniz Calculus Controversy, 1708–1730". in Schliesser, Eric; Smeenk, Chris. The Oxford Handbook of Newton. Oxford Handbooks. Oxford University Press. doi:10.1093/oxfordhb/9780199930418.013.9. ISBN 978-0-19-993041-8. OCLC 975829354. https://core.ac.uk/download/pdf/187993169.pdf. Retrieved February 9, 2024. 
  37. ↑ O'Connor, J. J.; Robertson, E. F. "Leonhard Euler". MacTutor. Scotland, UK: University of St Andrews. وقت November 9, 2022 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل February 9, 2024.  Unknown parameter |url-status= ignored (مدد)
  38. ↑ "Calculus (Differential and Integral Calculus with Examples)". Byju's. حاصل ڪيل June 13, 2024. 
  39. ↑ Franklin, James (July 2017). "Discrete and Continuous: A Fundamental Dichotomy in Mathematics". Journal of Humanistic Mathematics 7 (2): 355–378. doi:10.5642/jhummath.201702.18. ISSN 2159-8118. OCLC 700943261. https://scholarship.claremont.edu/cgi/viewcontent.cgi?article=1334&context=jhm. Retrieved February 9, 2024. 
  40. ↑ Maurer, Stephen B. (1997). "[[[:سانچو:GBurl]] What is Discrete Mathematics? The Many Answers]". in Rosenstein, Joseph G.; Franzblau, Deborah S.; Roberts, Fred S.. Discrete Mathematics in the Schools. DIMACS: Series in Discrete Mathematics and Theoretical Computer Science. 36. American Mathematical Society. pp. 121–124. doi:10.1090/dimacs/036/13. ISBN 0-8218-0448-0. OCLC 37141146. سانچو:GBurl. Retrieved February 9, 2024. 
  41. ↑ Hales, Thomas C. (2014). "[[[:سانچو:GBurl]] Turing's Legacy: Developments from Turing's Ideas in Logic]". in Downey, Rod. Turing's Legacy. Lecture Notes in Logic. 42. Cambridge University Press. pp. 260–261. doi:10.1017/CBO9781107338579.001. ISBN 978-1-107-04348-0. OCLC 867717052. سانچو:GBurl. Retrieved February 9, 2024. 
  42. ↑ Sipser, Michael. The History and Status of the P versus NP Question. STOC '92: Proceedings of the twenty-fourth annual ACM symposium on Theory of Computing. صفحا. 603–618. doi:10.1145/129712.129771.  Unknown parameter |s2cid= ignored (مدد)
  43. ↑ Ewald, William. "The Emergence of First-Order Logic". Stanford Encyclopedia of Philosophy. ISSN 1095-5054. LCCN sn97004494. OCLC 37550526. حاصل ڪيل June 14, 2024. 
  44. ↑ Ferreirós, José. "The Early Development of Set Theory". Stanford Encyclopedia of Philosophy. ISSN 1095-5054. LCCN sn97004494. OCLC 37550526. حاصل ڪيل June 14, 2024.  Unknown parameter |orig-date= ignored (مدد)
  45. ↑ Ferreirós, José (December 2001). "The Road to Modern Logic—An Interpretation". The Bulletin of Symbolic Logic 7 (4): 441–484. doi:10.2307/2687794. ISSN 1079-8986. OCLC 31616719. https://idus.us.es/xmlui/bitstream/11441/38373/1/The%20road%20to%20modern%20logic.pdf. Retrieved June 14, 2024. 
  46. ↑ Wolchover, Natalie (وڪي نويس.). "Dispute over Infinity Divides Mathematicians". Quanta Magazine. حاصل ڪيل June 14, 2024. 
  47. ↑ Zhuang, Chaohui. "Wittgenstein's analysis on Cantor's diagonal argument" (DOC). PhilArchive. حاصل ڪيل June 14, 2024. 
  48. ↑ Tanswell, Fenner Stanley (2024). Mathematical Rigour and Informal Proof. Cambridge Elements in the Philosophy of Mathematics. Cambridge University Press. doi:10.1017/9781009325110. ISBN 978-1-00-949438-0. OCLC 1418750041. 
  49. ↑ Avigad, Jeremy; Reck, Erich H. ""Clarifying the nature of the infinite": the development of metamathematics and proof theory" (PDF). Carnegie Mellon University. حاصل ڪيل June 14, 2024. 
  50. ↑ Warner, Evan. "Splash Talk: The Foundational Crisis of Mathematics" (PDF). Columbia University. وقت March 22, 2023 تي اصل (PDF) کان آرڪائيو ٿيل. حاصل ڪيل February 3, 2024.  Unknown parameter |url-status= ignored (مدد)
  51. ↑ Hamilton, Alan G. (1982). [[[:سانچو:GBurl]] Numbers, Sets and Axioms: The Apparatus of Mathematics]. Cambridge University Press. pp. 3–4. ISBN 978-0-521-28761-6. سانچو:GBurl. Retrieved November 12, 2022. 
  52. ↑ Snapper, Ernst (September 1979). "The Three Crises in Mathematics: Logicism, Intuitionism, and Formalism". Mathematics Magazine 52 (4): 207–216. doi:10.2307/2689412. ISSN 0025-570X. 
  53. ↑ Raatikainen, Panu (October 2005). "On the Philosophical Relevance of Gödel's Incompleteness Theorems". Revue Internationale de Philosophie 59 (4): 513–534. doi:10.3917/rip.234.0513. https://www.cairn.info/revue-internationale-de-philosophie-2005-4-page-513.htm. Retrieved November 12, 2022. 
  54. ↑ Moschovakis, Joan. "Intuitionistic Logic". Stanford Encyclopedia of Philosophy. وقت December 16, 2022 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل November 12, 2022.  Unknown parameter |url-status= ignored (مدد)
  55. ↑ McCarty, Charles (2006). "At the Heart of Analysis: Intuitionism and Philosophy". Philosophia Scientiæ, Cahier spécial 6: 81–94. doi:10.4000/philosophiascientiae.411. 
  56. ↑ Halpern, Joseph; Harper, Robert; Immerman, Neil; Kolaitis, Phokion; Vardi, Moshe; Vianu, Victor. "On the Unusual Effectiveness of Logic in Computer Science" (PDF). وقت March 3, 2021 تي اصل (PDF) کان آرڪائيو ٿيل. حاصل ڪيل January 15, 2021.  Unknown parameter |url-status= ignored (مدد)
  57. ↑ Rouaud, Mathieu (April 2017). Probability, Statistics and Estimation. p. 10. http://www.incertitudes.fr/book.pdf. Retrieved February 13, 2024. 
  58. ↑ Rao, C. Radhakrishna (1997). Statistics and Truth: Putting Chance to Work (2nd ed.). World Scientific. pp. 3–17, 63–70. ISBN 981-02-3111-3. OCLC 36597731. 
  59. ↑ Rao, C. Radhakrishna (1981). "Foreword". in Arthanari, T.S.; Dodge, Yadolah. Mathematical programming in statistics. Wiley Series in Probability and Mathematical Statistics. New York: Wiley. pp. vii–viii. ISBN 978-0-471-08073-2. OCLC 6707805. 
  60. ↑ Whittle 199410–11, 14–18.
  61. ↑ Marchuk, Gurii Ivanovich. "G I Marchuk's plenary: ICM 1970". MacTutor. School of Mathematics and Statistics, University of St Andrews, Scotland. وقت November 13, 2022 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل November 13, 2022.  Unknown parameter |url-status= ignored (مدد)
  62. ↑ Johnson, Gary M.; Cavallini, John S. Phua, Kang Hoh; Loe, Kia Fock, وڪي نويس. [[[:سانچو:GBurl]] Grand Challenges, High Performance Computing, and Computational Science] Check |url= value (مدد). Singapore Supercomputing Conference'90: Supercomputing For Strategic Advantage. World Scientific. صفحو. 28. LCCN 91018998. حاصل ڪيل November 13, 2022. 
  63. ↑ Trefethen, Lloyd N. (2008). "Numerical Analysis". in Gowers, Timothy; Barrow-Green, June; Leader, Imre. The Princeton Companion to Mathematics. Princeton University Press. pp. 604–615. ISBN 978-0-691-11880-2. OCLC 227205932. http://people.maths.ox.ac.uk/trefethen/NAessay.pdf. Retrieved February 15, 2024. 
  64. ↑ Monastyrsky 20011: "The Fields Medal is now indisputably the best known and most influential award in mathematics."
  65. ↑ Riehm 2002778–782.
  66. ↑ "Fields Medal | International Mathematical Union (IMU)". www.mathunion.org. وقت December 26, 2018 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل February 21, 2022.  Unknown parameter |url-status= ignored (مدد)
  67. ↑ 67.0 67.1 "Fields Medal". Maths History. وقت March 22, 2019 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل February 21, 2022.  Unknown parameter |url-status= ignored (مدد)
  68. ↑ "Honours/Prizes Index". MacTutor History of Mathematics Archive. وقت December 17, 2021 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل February 20, 2023.  Unknown parameter |url-status= ignored (مدد)
  69. ↑ "About the Abel Prize". The Abel Prize. وقت April 14, 2022 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل January 23, 2022.  Unknown parameter |url-status= ignored (مدد)
  70. ↑ "Abel Prize | mathematics award". Encyclopedia Britannica. وقت January 26, 2020 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل January 23, 2022.  Unknown parameter |url-status= ignored (مدد)
  71. ↑ "Chern Medal Award" (PDF). www.mathunion.org. وقت June 17, 2009 تي اصل (PDF) کان آرڪائيو ٿيل. حاصل ڪيل February 21, 2022.  Unknown parameter |url-status= ignored (مدد)
  72. ↑ "Chern Medal Award". International Mathematical Union (IMU). وقت August 25, 2010 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل January 23, 2022.  Unknown parameter |url-status= ignored (مدد)
  73. ↑ "The Leroy P Steele Prize of the AMS". School of Mathematics and Statistics, University of St Andrews, Scotland. وقت November 17, 2022 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل November 17, 2022.  Unknown parameter |url-status= ignored (مدد)
  74. ↑ Chern, S. S.; Hirzebruch, F. (September 2000) (en ۾). Wolf Prize in Mathematics. doi:10.1142/4149. ISBN 978-981-02-3945-9. https://www.worldscientific.com/worldscibooks/10.1142/4149. Retrieved February 21, 2022. 
  75. ↑ "The Wolf Prize". Wolf Foundation. وقت January 12, 2020 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل January 23, 2022.  Unknown parameter |url-status= ignored (مدد)
  76. ↑ 76.0 76.1 "Hilbert's Problems: 23 and Math". Simons Foundation. وقت January 23, 2022 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل January 23, 2022.  Unknown parameter |url-status= ignored (مدد)
  77. ↑ Feferman, Solomon (1998). "Deciding the undecidable: Wrestling with Hilbert's problems". [[[:سانچو:GBurl]] In the Light of Logic]. Logic and Computation in Philosophy series. Oxford University Press. pp. 3–27. ISBN 978-0-19-508030-8. https://math.stanford.edu/~feferman/papers/deciding.pdf. Retrieved November 29, 2022. 
  78. ↑ "The Millennium Prize Problems". Clay Mathematics Institute. وقت July 3, 2015 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل January 23, 2022.  Unknown parameter |url-status= ignored (مدد)
  79. ↑ "Millennium Problems". Clay Mathematics Institute. وقت December 20, 2018 تي اصل کان آرڪائيو ٿيل. حاصل ڪيل January 23, 2022.  Unknown parameter |url-status= ignored (مدد)


حوالي جي چڪ: "lower-alpha" نالي جي حوالن جي لاءِ ٽيگ <ref> آهن، پر لاڳاپيل ٽيگ <references group="lower-alpha"/> نہ مليو

"https://sd.wikipedia.org/w/index.php?title=رياضي&oldid=298314" تان ورتل